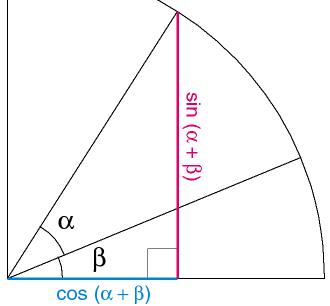
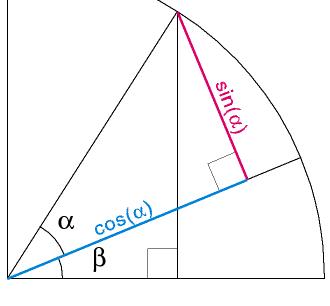
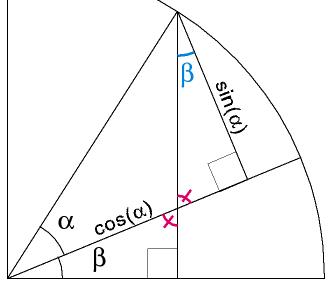
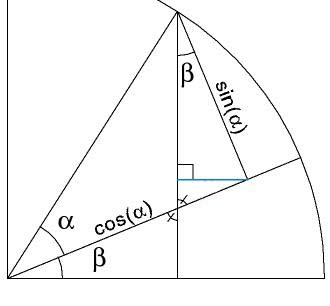
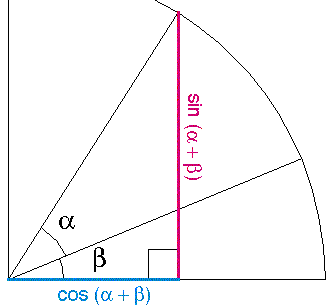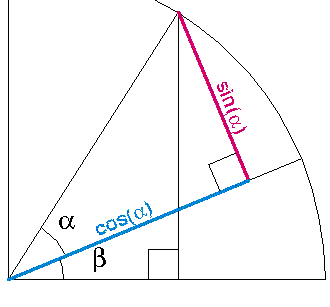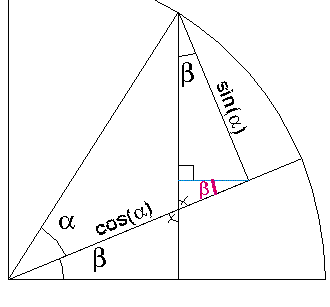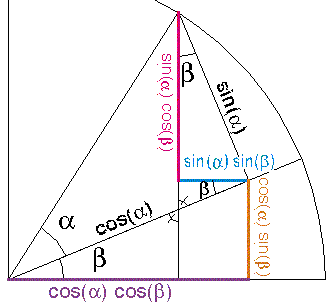
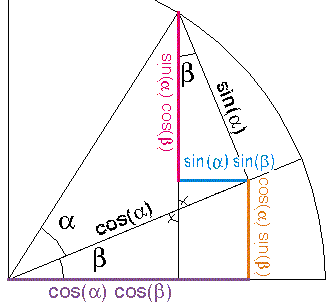
 |  |
|
First two frames use definition of cos and sin (circle is unit circle) 3rd frame: opposite angles are equal, Angles of a triangle add up to 180 therefore top angle of upper right triangle is Beta 4th frame: Erect a perpendicular off of sin(alpha) line - this makes a small right triangle whose right most angle is Beta (because this angle added to the angle opposite beta = 90.
| cos(a+b) = cos(a) cos(b) - sin(a) sin(b)
and sin(a+b) = sin(a) cos(b) + sin (b) cos(a) |
 |  |
|
| Step 2: Definition of sine and cosine |
 |
 |
| Step 3: Opposite angles are equal, triangle angles sum to 180 degrees |
Step 4: Erect a perpendicular from the vertical line. |
| Step 5 (to the right): From step 4 see the upper tirangle whose hypotenuse is sin(alpha) The side opposite angle beta is hypotenuse * sin(beta) or sin(alpha) * sin(beta) The leg adjacent to beta of the same triangle is hypotenuse * cos(beta) or sin(alpha) * cos(beta) - Then the triangle at the bottom with purple and orange legs has hypotenuse of cos(alpha). It's legs are cos(alpha) * cos(beta) (purple leg) and cos(alpha) * sin(beta) (orange leg). - Adding the red and orange lines gives the vertical distance and subtracting the green from the purple line gives the horizontal distance. |
|
| Hop David Coloring Books |
 |
| A Hop David coloring book of Escher like tessellations |
 |
| A Hop David coloring book of various geometrical landscapes. To be released in February of 2009. A lot of pages are devoted to space filling polyhedra with an emphasis on octahedra alternating with tetrahedra. There's also some polyhedra studies, logarithmic spirals, fractals (only the first few iterations or they'd be impossible to color!) and some miscellaneous. This book includes a CD to print out pages. I think these coloring books will be a good tool for teachers in helping get kids interested in math. |